The Lunes of Hippocrates

A Short Introduction and the Latest Discoveries:

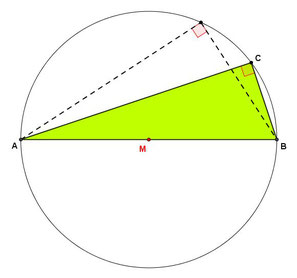
1.) The first figure shows Thales' theorem:

A, B and C are points on a circle.

If line segment AB is the diameter of this circle,

then the angle at C is always a right angle.

2.) The second graphic shows the Pythagorean theorem:
The sum of the areas of the two squares on the legs of a right triangle

equals the area of the square on the hypotenuse.

Surprisingly the forms don't have to be squares. It works for all similar

figures, like half squares or semicircles (… as shown).

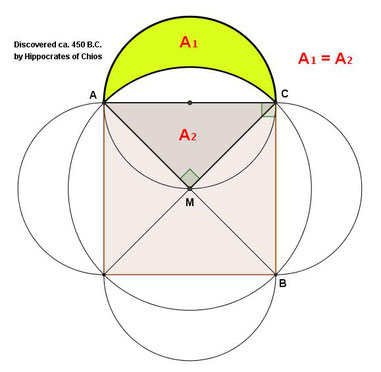
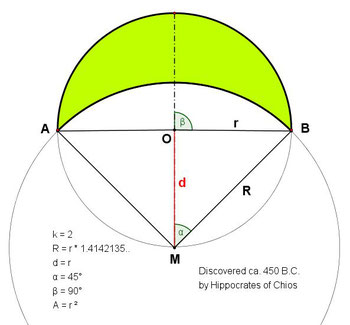
3.) The next figure shows the so-called Lunes of Hippocrates,

named after Hippocrates of Chios (not the physician!)

who lived about 450 B.C.

(Sometimes they are also called Lunes of Alhazen,

named after an Arab mathematician of the 10th and 11th century.)

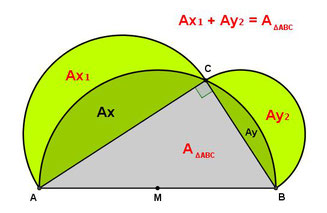
If the great semicircle on the hypotenuse is folded up,

two circle segments (Ax and Ay) appear, which overlap with the semicircles.

Eliminate the circle segments and you get two lunes (Ax1 and Ay2),

which have the same area as the right triangle ABC.

4.) In the next graphic you see a special case:

If the right triangle ABC is also isosceles,

then one lune has the same area as

the half triangle ABC (...which is triangle AMC).

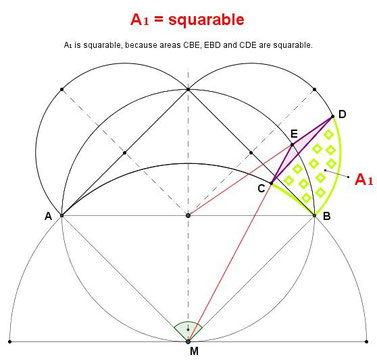
This lune can therefore be transformed into a square,

and that only with compass and straightedge.

In the following we call this feature of a lune "squarable".

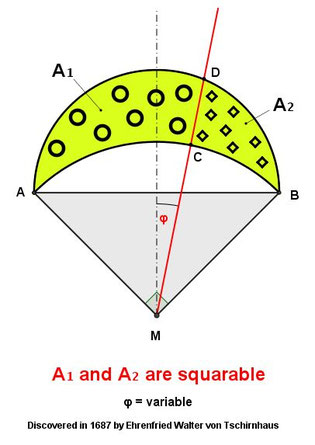
5.) Tschirnhaus discovered in 1687 the following phenomenon:

You can draw a line through the center M and cut the lune into two parts.

These two parts are also squarable.

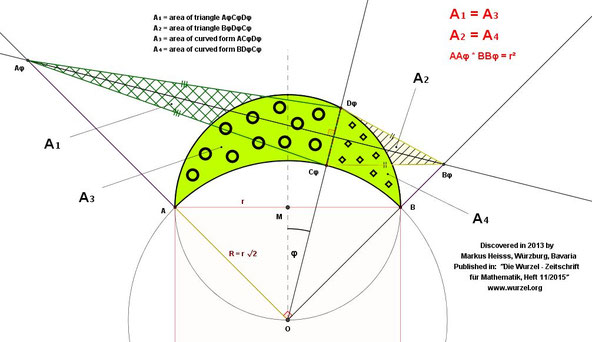
6.) Heisss [sic!] discovered in 2013 an easy construction

to square these two parts of the lune.

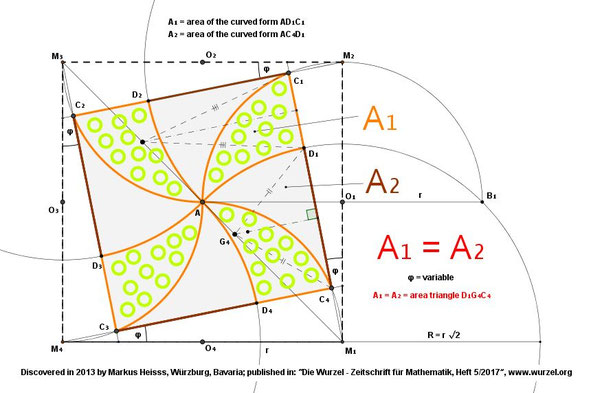
7.) Another remarkable discovery by Heisss in 2013 was a figure

that looks like a wind wheel.

You can see two different forms of lune fragments,

which have the same area!

Both are therefore simply squarable.

8.) It is possible to lay two or more lunes (of the right size) together.

If you lay eight lunes in this way together,

you can see a figure like the coiled shell of a snail.

The eight lune fragments fill an angle of 360° at point B.

In the end you get a curved squarable wedge with 0°,

which looks relatively similar to the wedge in picture 9.

9.) The curved wedge GCF can also simply be squared.

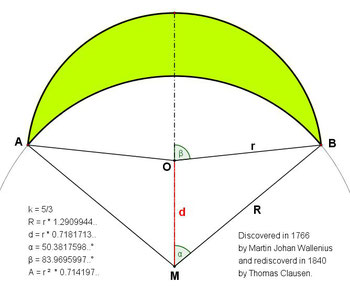
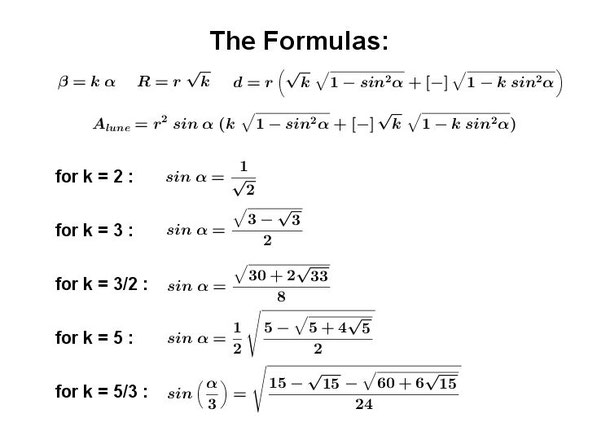
10) Just for completeness:

Nikolai Chebotaryov and Anatoly Dorodnov prooved in 1947,

that there are exactly five squarable lunes.

Three were discovered by Hippocrates himself,

and the last two were found in the year 1766 by Martin Johan Wallenius,

and rediscovered in 1840 by Thomas Clausen.

You can draw the lunes simply with two circles

and the distance d of their centers.

(Circle 1 with center O and radius r and circle 2 with center M and radius R.)

11) Of course it is possible to square portions of these five lunes:

Divide both arcs into an equal number of sections

and join the points as shown below.

Sadly, the elegant method of Heisss doesn't work for the other four lunes,

because the (red) lines don't intersect in one common point!

12) I hope you enjoyed it!

Or would you like to see more?  ==>  [here]

References and further information:

1. Book:  ==>  Thomas Heath: "A History of Greek Mathematics", Volume I, p.200, Dover Publications, 1981

2. Website:  https://markus-heisss.jimdofree.com/geometrie-handskizzen/
   
3. Website:  https://en.wikipedia.org/wiki/Lune_of_Hippocrates

4. Magazine: "Die Wurzel – Zeitschrift für Mathematik", Heft 11/2015, p.234, "Die Begradigung eines Möndchens" ==> www.wurzel.org

5. Website:  http://www.gogeometry.com/school-college/4/p1335-squaring-circle-kite-lune-mobile-apps.htm
6. Website:  https://triangle-geometry.jimdofree.com/lune-of-hippocrates-2/